Kola s čelním ozubením

Wheels with spur gearing

Nejlepší způsob výroby ozubených kol je pomocí odvalovky. Pro stolní soustruhy ji vyrábí za pár peněz pan Vávra, viz jeho stránky. Když jsem ji sháněl, ještě nebyla v prodeji, tak jsem sáhnul po tvarových frézách.

The cog-wheels are best made on the spur-geat hob. For the mini lathe it make Mr. Vavra, see his pages. It didn't for sale when I would, so I bought a shaped cutters.

Tuhle sadu jsem koupil u M&V, evolventní frézy najdete tady. Nebyla to žádná láce, ale dělá se s nimi snadno a rychle. Pro každý modul (viz dále) je jiná sada.

This set I bought from M&V, evolvent cutters are here. It's a little expensive, but it works easy and fast. For every module (see next) is another set.

Tady je dobře vidět tvar zubů, který odpovídá mezeře mezi zuby kola. Pro různé počty zubů (různé průměry kola) jsou jiné tvary fréz, proto se prodávají v sadách.

A shape of tooth is good to see on this picture. The shape is equal space between tooths of wheel. For different number of the tooths (different wheel diameter) is different cutter shape. That is why is sold in sets.

Takže dotknout se frézou polotovaru, zajet do záběru o výšku zubu, sem a tam, pootočit, sem a tam, pootočit a pořád dokola.

So, touch by cutter to semi-product, go to stroke for a tooth high, forward and back, rotate, forward and back, rotate and so on.

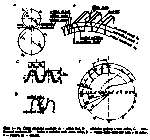
Modul je číslo, odpovídající velikosti ozubení (a fréz). Průměr roztečné (střední) kružnice = počet zubů kola * modul. Výška zubu = hloubka záběru frézy = 2.25 * modul.

Module is the number that respond of tooth size. Diameter of the pitch-circle = number of tooth * module. High of tooth = stroke of cutter = 2.25 * module.

Průměr polotovaru = průměr roztečné kružnice + 2 * modul. Úhel otočení mezi zuby = 360 / počet zubů. Rozteč os dvou kol je součet poloměrů roztečných kružnic. To je vše.

Diameter of semi-product = diameter of the pitch-circle + 2 * module. Angle of rotate semi-product between tooths = 360 degree / number of tooths. Pitch of two wheels is sum of this wheels pitch-circle radiuses.

Kuželová ozubená kola

Conic gearing wheels

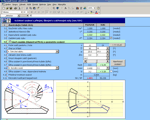
Výpočet kuželových kol je složitý, protože všechny rozměry a úhly se mění s velikostí i s převodovým poměrem kol. Firma MITCalc, prodává programy na výpočet kol, ale i demoverze je použitelná. Zde je přímo excelovská tabulka s výpočtem.

Calculate of conic gearing wheels is too complex. I find software for this wheels here (in English).

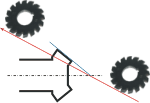
S jistou nepřesností lze vyrobit i kuželová kola pomocí frézy. Všimněte si rozdílného úhlu hlavy zubu (= povrch polotovaru, zelená čára) a úhlu paty (= cesta frézy, červená čára). Tím se simuluje kuželovitý tvar zubu. Díky změně zanoření frézy se i mezera mezi zuby rozšiřuje.

With some inaccuracy is possible to make conic gearing wheels by shape cutter. Notice that angles of top of tooth (=surface of semi-product, green line) and bottom of tooth (=track of cutter, red line) are different. This simulate conic shape of tooth.

Zde je vidět, jak se šířka mezery a zubu mění. Nevychází to úplně přesně, tvat zubu se mění špatně, ale i tak tyto převody po okartáčování chodí docela hladce.

There is good to see variable width of tooths. It's not fully accurate but it works very good on my models.

Záběr frézy do pastorku.

Go to stroke.

Zde je vidět natočení rotačního stolu oproti směru posuvu.

There is good to see turning of rotating table against mill table.

Hotová nádhera.

Finished wonder.